...vWe extend the precedent group to a four-components orthochron set. This operation gives a geometrical interpretation of antimatter after Dirac.

1) Introduction :

...In a former paper [1] we have presented a description of elementary particles ins a ten-dimensional space, i.e. space-time (x,y,z,t) plus six additional dimensions :

(1)

 
We presented a 16-dimensions group, an extension of the Poincaré orthochron subgroup, acting on :

- its 16-dimensions momentum space

- its 10-dimensional movement space.

The six additional components of the momentum have been identified to the charges of the particles :

(2)

so that the momentum becomes :

(3)

(4)

after J.M.Souriau [1].

We have figured the link between the species of moments and the species of movement, suggesting that :

- The movement of matter corresponds to { z ⁱ > 0 } sector.

- The movement of antimatter corresponds to { z ⁱ < 0 } sector.

- The movement of photons corresponds to { z ⁱ = 0 } plane.

 
All that must be now justified.

2) Introducing a four components group. Geometrization of Dirac's antimatter.

...The precedent 16-dimensional group had two components, correspondong to the two orthochron components of the Lorentz group, L_n ( neutral component ) and L_s , with :

(5)

Our group was an extension of the orthochron Poincaré sub-group :

(6)

and we wrote it :

(7)

The corresponding coadjoint action was :

(8)

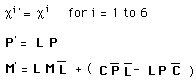
with :

(9)

...In such a group no element transforms the movement of a matter mass-point into the movement of an antimatter mass-point, and vice versa. According to the chosen definition of antimatter, through a :

(10)

some element should reverse the additional dimensions. With :

(11)

 
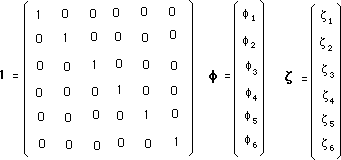
we can write the precedent group into a more compact form :

(12)

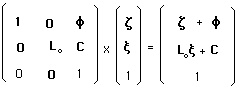
It contains the neutral element :

(13)

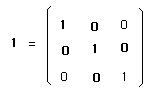
The matrix that reverses the additional dimensions is be the following orthochron commuter :

(14) 

 
We can duplicate the precedent group through the operation :

(15)

It is equivalent to write the new four component group, whose element is :

(16)

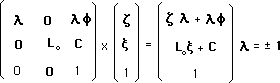
The corresponding coadjoint action is :

(17)

We see that ( l = - 1 ) reverses the charges. In that case the inversion of the additional dimensions :

(18)

 
goes with a :
(19)

 
which corresponds to Dirac's description of antimatter [4], so that the present paper represents a geometrization of antimatter after Dirac.